Forgery Drugs, Health and Development

Diana Loubaki^*, Moyo N. Zololo

Economics Department, University Marien N’Gouabi, Brazzaville, Congo

Abstract

The aim of this article is to study the impact of forgery drugs on health in Sub-Saharan-Africa. An endogenous growth model which supports health is used to conduct the study. We found that, forgery drugs business and corruption are linked since it yields as more profit as original drugs do at cheaper investments, since consumers lack information able to help them identify forgery with original in the decision to buy drug is unknown since the market is mostly endowed with piracy products. Indeed, health becomes a random variable which recovery becomes a deal. In that context, sustainable development path is difficult to be reached through the market alone. The social planner establishes optimal health state levels in order to tax the drug business firm for forgery drugs provided to consumers to achieve a Pareto optimality in health state. None study on growth which supports health has studied forgery drugs impact on health before that model.

Keywords

Forgery Drug, Original Drug, Corruption, Development Sustainability, Health

Received: August 9, 2015
Accepted: August 26, 2015
Published online: September 2, 2015

@ 2015 The Authors. Published by American Institute of Science. This Open Access article is under the CC BY-NC license. http://creativecommons.org/licenses/by-nc/4.0/

1. Introduction

Forgery is extremely old and it is difficult to precise its beginnings. The problem began to emerge by 1780 with the development of labels. Therefore, in 1789, the term of "forgery" was introduced to qualify it as the action which consists on copying or imitate something without having the license required, or being the owner of the product and equivalently, to pretend that it is original. Consequently, it is the violation of the property right. Forgery can be seen in almost all the fields actually like in industrial production, in musical copies of the original, in clothes high quality labels, luxury accessories such as fragrance, bags, food production, historical objects, drugs,…With the globalization, the increase of good exchanges among countries is keeping increasing both in quantity and in quality. Therefore, increases also risks that forgery be considered as real products if the information hold on the product is not good enough. The problem is relevant when concerning drugs because of health troubles specifically in the concern of Sub-Saharan Africa where products departure origins as well as quality, are not controlled at all. Indeed, the market sells both forgery and original drug products which improvements on health state become a random event. For specific illness greatly met in Africa such as HIV/AIDS i.e there are 68% of total infected people in the whole world, therefore forgery drugs distribution in the market, deserve more attention in order to understand what can be done to fight against this phenomenon to maintain population health state in balanced which is also a component of growth and development. Both according to World Health Organization and the intellectual property rights, in Angola and in Congo, 46% of drugs are forgery drugs among them, 10.6% of forgery drugs are provided by seller with license against 90% of forgery drugs provided by sellers without license to sell drugs Gallup¹ surveys show counterfeit drugs are widespread in sub-Saharan Africa. In Cameroon, 70% of the whole drugs are forgery drugs, the same thing in Burkina Faso and in Angola. Despite of that, there doesn’t exist, studies which try to analyze the impact of forgery drugs on health in Sub-Saharan Africa. Consequently, the aim of this article is to study the impact of forgery drugs in health state in the purpose of development sustainability improvements in Sub-Saharan Africa. The article uses the literature of growth which support health in order to look for the way sustainable development path can emerge from that given situation i.e forgery drugs introduction in the market.

The model is a theory of economic development where exist consumers who work consumes goods and drugs. But drugs are mostly piracy products and sometimes are original products. The drug seller business employs corrupted agents who negotiate with imitator on the entry of forgery drugs for pecuniary gains, but the non corrupted agents don’t do that. Therefore, if the proportion of forgery drugs are too high compare to original drugs, health recovery can’t be achieved, the economy converge to an under development path with low growth and low health states. Otherwise if original drugs are higher than forgery drugs, development can emerge described by an inverted U-shape curve. The random character of life duration introduced by forgery drugs in the market and consumers claim on losses on their utility function, leads the social planner introduces a tax on corrupted activity in order to reach sustainable development path and make corrupted agents cease their activity which maintain under development in the poor country (See the literature evocated on the notes).²

The scientific contribution of the model holds on three aspects: first the introduction of forgery drugs in the endogenous growth model which support health, second, the non intentional will for agents to buy forgery drugs for health recovery, third, the introduction of corruption in exchange trade based on drug goods.

According to the literature of economic growth, from 1950s to 1960s, economists assume technological progress to occur in an unexplained manner called exogenous (Harrod-Domar, 1948; Solow, 1956). In the 1960s, growth theory consisted mainly of the neoclassical model based on the optimal saving methodology developed by Ramsey (1928), like Solow (1956), Swan (1956), Cass (1965), and Koopmans (1965). One feature of those models is the convergence property generated. The lower the starting level of real per capita gross domestic product (GDP) the higher is the predicted growth rate. But those predictions were not confirmed by empirical investigations nor by observations, thus there where a serious problem that the theory was unable to explain until the mid 1980s, since then, the research on economic growth experienced a boom, beginning with the work of Romer (1986). The new endogenous growth theories focused now on productivity advances that derive from technological progress and from human capital in the form of education (Lucas, 1988; Barro and Sala-i-Martin (1995) in order to explain the differences among countries in the world. These theories also explore extensions to allow for open economies, diffusion of technology (Aghion-Howit, 1992), migration of persons (Docquier-Rapoport, 2012), fertility choice (Galor and Weil, 2000)), and variable labor supply (Lucas, 1988). The government can be important in the models in terms of its policies on maintenance of property rights, encouragement of free markets, taxation, education, and health. The concept of capital in the neoclassical model can be usefully broadened from physical goods to include human capital in the forms of education, experience, goods as well as health (Lucas, 1988; Stockey, 1988; Rebelo, 1991; Caballe and Santos, 1993; Mulligan and Sala-i-Martin, 1993; Barro and Sala-i-Martin, 1995a.). One area that has received little attention in the recent literature on growth theory is the relationship between health and economic growth³. Two preliminary efforts in this direction are Ehrlich and Lui (1991) and Meltzer (1995). Also, the empirical work of Barro (1996) and others suggests that health status, as measured by life expectancy or analogous aggregate indicators, is an important contributor to subsequent growth. In fact, initial health seems to be a better predictor than initial education of subsequent economic growth. Recent work on endogenous growth theory applies, in particular, to discoveries of medicines or medical procedures (Romer, 1986; Lucas, 1988; Rebelo, 1991)⁴. The government therefore has great potential for good or ill through its influence on the long-term rate of growth. The population's overall health status is measured by the log of life expectancy at birth at the start of each period. Until now, it can’t be find analysis focused on the adverse effects of disease i.e the severity of the disability in comparison with loss of life. Indeed, this model focuses on the adverse effects of forgery drugs on health, which is for instance not really known. Whereas empirical existing work on the subject uses some indicators without going farer through the proposition of a real economic policy to close the debate, we only can find studies like Rosen (1988) health status index by life expectancy at birth impact on economic growth. Previous theoretical work on growth has often stressed the role of education as a contributor to human capital and economic development (Azariadis-Drazen, 1990) but has tended to neglect the role of health. Indeed, Barro (2013) describes a framework as an extension of the neoclassical growth model to incorporate a concept of health as a capital. The results found are: better health tends to enhance economic growth. At the same time, advances in economic performance encourage further accumulation of health capital. The model includes a direct impact of health on productivity. That is, for given quantities of labor hours, physical capital, and worker schooling and experience, an improvement in health raises a worker's productivity. In addition to this direct effect, an improvement in health lowers rates of mortality and disease and thereby decreases the effective rate of depreciation on human capital; that is, on schooling and health itself. Through this channel, an increase in health raises the demand for human capital and thereby has a further, indirect positive effect on productivity. Therefore, the link between health and growth is positive since it increases life expectancy and ability at work. Consequently may also lead economic performance increase in developing countries? In the prospect of development take-off, according to the growth literature presented, health plays a crucial role in that purpose. Unfortunately in developing countries and specifically in Sub-Saharan Africa, forgery drugs are introduced in the market, so that the results expected for the role of health in productivity gain can’t fully be obtained. The study introduces corruption in drug exchange trade, thus we give some details on the literature of corruption.

The literature of corruption can be summarized in three approaches. The first research line addresses why policymakers, business leaders, and private citizens should concern themselves with the existence and elimination of corruption (Qian, R., 2012). This research program focuses on the effect of policies and reforms on growth and macroeconomic stability and concludes that differences across countries in the quality of their institutions explain growth heterogeneity. The second research line addresses how and when political, social, and economic forces contribute in counter corruption drives, cost-benefit analysis on the part of a country’s leaders in order to determinate existence or lack of an official policy (Banuri. and Eckel, 2012). The third approach focuses on what types of policies effectively counter corruption and highlight effective anticorruption instruments. In a formal way, economic models the corrupt employee as a rational actor who decides whether to engage in corrupt activity by balancing the potential benefits against potential costs and consequences. Therefore, policies that worsen the consequences of engaging in corruption by increasing severity of punishment and likelihood of detection will lower corrupt activity. Bardhan (1996) argues that the frequency of corruption in a society changes the balance of its marginal costs and benefits for an official.

Consequently, the aim of this article is to study the impact of forgery drugs on health in an endogenous growth model in order to highlight negative effects which may slow development sustainability occurrence. The solution proposed to eradicate the problem is based on an optimal program done by the government to define health state thresholds as well as corrupted firms of agents through a tax on non respect of the law.

The article is organized as follow: section 2 setups the model of health and forgery drugs, section 3 provides results, section4, provides discussion and section 5 concludes on the analysis.

2. The Endogenous Growth Model of Health and Forgery Drugs

Consider a developing country where exist two firms and a constant labour force, L(t) at each period, divided in two parts such that L(t)=L^Y(t)+L^D(t) where L^Y(t) works in the firm which produces an homogenous consumption good and the other labour force part, L^D(t) is employed in the other firm which sell drugs provided from abroad countries.

2.1. Good and Drug Business Production Functions

The good production sector employs a stock of labour force, L^Y(t) with a physical capital stock, K(t) to produce consumption goods using a neoclassical function of Cobb-Douglas expressed by equation (1) i.e

                         (1)

Where  is the inputs elasticity parameter and A is the productivity parameter of the consumption good production sector.

Rewriting the production function (1) in intensive form, setting k(t)=K(t)/L^Y(t) and y₁(t)=Y₁(t)/L^Y(t), then per capita production function of the consumption goods is expressed by (2) i.e

                               (2)

Following Romer (1990) on the basis of Dixit-Stiglitz (1977), there exist N pharmaceutical goods or drugs indexed by i such that, x=(x_i)_1<i<∞ is the stock of drug list available in the country, but we don’t have exact information on the quality of drugs provided by each country indexed by i because there is no public control on their entry inside the poor country. Therefore, there exist forgery drugs among the product available which create uncertainty on drug action on health, optimal health is hard to be reached on that context. Therefore, the production function of the drug seller company is written with pharmaceutical goods available in the poor country, such that

               (3)

Where E is the productivity parameter of the drug seller business, B_i is the efficiency of the drug i in health action, β is the drug seller business elasticity parameter. Each good comes from a given country i, thus x(i) is the stock of drug i. For simplicity on the function, we assume all the drugs to be the same kind i.e homogenous goods with quality differentials apparently, indeed the whole stock of drug is finite, D= qD+pD where p is a proportion of high original drugs and q is a proportion of forgery drugs, where p+q=1 highlights the quantity of the whole drug of market. For simplicity of the analytical solution, we assume, x_i=x, therefore, we can write equation (3) such that equation (4) i.e

                       (4)

Rewriting the production function (4) in intensive form such that, and y₂(t)=Y₂(t)/L^D(t), then per capita production function of the drug goods is expressed by (5) i.e

                                 (5)

Where h(t)=DB/L^Y(t)

Profit maximization yields the standard first order conditions i.e the wage rate income equals the marginal product of the good production, sector, w^Y(t) and the drug sector, w^D(t) respectively expressed by (6) and (7)

                        (6)

                              (7)

The stock of drugs is assumes to be a capital (Romer, 1990). Therefore, each firm of the both categories determinate its own interest rate when maximizing its profit which we denote  respectively by, r₁ for the production sector and by, r₂ for the drug business sector where r₁≠r₂ i.e

                                   (8)

                           (9)

If r₁(t)=r₂(t) , it yields the equilibrium among the both firms of the country, it is the equality in marginal capital productivity, thus equality in profits term.

If r₁(t)<r₂(t) , we join Solow neoclassical result with respect to absolute convergence where marginal benefit of per-capita capital stock, k(t) is higher than that of per-capita capital stock h(t),. Thus, with technological improvements, the firm of good production grows faster than the drug seller business. In the long-run, firm1 grows faster than firm2, it is the absolute convergence notion.

If r₁(t)≻r₂(t) , we join the AK result with respect to conditional convergence . The marginal benefit of per-capita capital stock, k(t) is lower than the marginal benefit of per-capita capital stock h(t). Thus, in this case, the drug seller business is stronger than the good consumption sector of production. In the long-run, the firms are not the same because the marginal benefit of capital is no more decreasing like in the previous case.

Assumption 1:

 yields

               (10)

2.2. Preferences

Following Stockey (1998), the agents share the same intertemporal utility function expressed over consumption, c(t) and health state, d(t) where the impact of forgery drugs in health state is captured by the parameter, γ inside [0,1] which mostly maintains the agent’s health under the normal level because of piracy of trades and corruption in drug entry in the frontier depending of its sign. Therefore, the utility function of the agent is expressed by equation (11) i.e

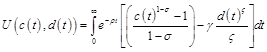    (11)

Where ρ>0 is the intertemporal discount rate, ζ is health alteration intensity and σ is the inverse of the elasticity of substitution. The country where live the agent sells drugs and a great part of them are not real, thus are forgery products mostly, which impact on health is captured by the parameter γ and intensify by the parameter, ζ. Therefore, if γ≻0, then the drug taken by the agent is not a real one or it is a forgery drug and highly damaged the utility function of the agent and can lead to the extinction of the agent existence if no action is quickly engaged. Otherwise, if γ<0, then the drug taken by the agent is a good substitute of the one produced in the developed country, it may be a real one, indeed the utility function of the agent is increased. Finally, if 0<γ<1, the impact on the utility function, depends on the level of γ such that if γ is high, the utility function of the agent is damaged, in contrast, if γ is low, the agent health state follows a good evolution which maintains his utility function at quite good level. The agent has a probability, ζ to consume a piracy drug or a forgery drug, which also has a negative impact on the utility function expressed by equation (11) depending on its level. As we know that 0<ζ <1, indeed, if ζ tends to 0, then the agent is not in a developing country and doesn’t face forgery drug risk, otherwise, if ζ tends to 1, then the agent lives in a poor country where trade goods departure country and quality are not under the control of public authority, thus face high probability of death since, first the drug taken has no positive effect on health, second, it makes γ increases, thus speeds health alteration and leads to utility function damage which cannot be viewed sooner, so that, it may lead the agent to the extinction of his life sooner. This reality may partly explain today, the low levels of life expectancy of the agents who live in poor countries because when ill, they die faster without living enough time for the drug action to play⁵.

Therefore, the social planner evaluates the impact of drug intake on health state of the economic agents through an optimal program in order to know more about it and find an appropriate policy for protection of the agents’ health with future drugs control yielding to a tax rate on forgery drugs distribution.

2.3. The Optimal Program

The social planner program aim is to evaluate the agents well being in regard to forgery drugs entry and acts on the basis of the results obtained to tax the forgery drugs seller who also are corrupted agents.

The budget constraints of the agents are expressed such that:  where j=Y, D

The optimal program consists on the resolution of the Hamiltonian which can be written such that:

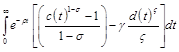=  + +     (12)

The first order conditions of the optimal program can be written such that:

                          (13)

                         (14)

Computing  establishes a link between per-capita physical capital and drug expressed by equation (14) i.e

                       (15)

3. Results

Proposition 1: the optimal program defines a couple of per-capita drug and physical capitals expressed such that equations (16) and (17) i.e

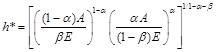        (16)

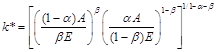        (17)

Proof: let per-capita physical capital given by equations (10) and (15) to be equal, then we obtain a system of two equations with two unknown variables (k*,h*), expressed by proposition 1.

Proposition 2: the optimal health state, d(t)=d* is a function of the quality of drug taken captured by the parameter,  and expressed such that

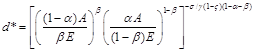     (18)

Proof

From equations (13), it can be established a link with optimal per-capita capital given by proposition 1 and using the logarithm function and viewing the neglect function around 0, then we obtain the optimal health state expression announced in proposition 2

Proposition 3: the positive mechanics of health state maintenance are: the drug seller products efficiency, E; high quality drug selection, β as well as the proportion of high quality drugs introduced in the market measured by, ζ

Definition 1: the sustainable growth path, g* is defined by the locus on the space where, r₁ =r₂ , w^D(t)=w^Y(t) such that, g*=k*/h*

Proposition 4: according to definition 1, the sustainable growth rate is expressed by equation (19) such that:

                              (19)

Indeed, the mechanics of economic growth are: the elasticity of per-capita physical capital and the elasticity of labor force of the drug business in regard to his honesty when trade with drug goods abroad. In contrast, the variety of drugs efficiency differential due to their quality differential may affect labor force, which turns out to be not efficient enough at work

Proof: applying definition 1 inside equation (10) and using proposition 1 result, yield the sustainable growth rate expressed by equation (19), differentiating the parameters in the growth and knowing the action of each parameter, we conclude to the proposition 4 results

4. Discussions

Because there exist a probability p for the drug to be original and a probability q for the drug not to be original, thus a forgery drug where p+q=1, indeed the drug seller company, also employs corrupt agents who leave forgery drug enter in the country and non corrupted agents who ensure drugs quality, thus drug action on health state can be written such that, d=pd+qd where p+q=1 and d expressed the impact of drugs on health state of a given agent.

4.1. First Discussions Category

If q=0, then corruption is absent, thus all the drugs are original. Therefore, there exist a unique optimal sustainable path, (d, g) respectively expressed by health state and development sustainability, such that d=d* and g=g*

If p=0, then all the drug sellers are corrupted agents, thus all the drugs are forgery, there exist a non optimal sustainable path, (d, g) respectively expressed by health state alteration and development retards, such that d<d* and g<g*. Therefore,  and  i.e the sustainable path is at its lowest state and the country is under developed.

If 0<p, q<1, then d≤d* and g≤g*, the sustainable development path can be reached if p is higher than q and close to 1, then d=d* and g=g*, since most of the drug sellers are not corrupted agents, the economy may reach its sustainable development path despite of some existing forgery drugs. Otherwise if p is lower than q such that it is quite close to 0, health may be highly damaged due to more forgery drugs than original drugs in the market. Therefore, the sustainable path can’t be reached because d<d* and g<g*.

4.2. Second Discussions Category, the Solution

Economic policy introduces a tax rate on forgery drugs which is supposed to be paid by the drug seller through controls and health state damages claims such that:  =p+q(1+s)=(1+sq) which yields:  =(1+qs)

Where s is the degree of corruption of the agent which also highlights how negotiations can vary in function of the expectation of the drug seller.

When q≠0 and p≠1, there exist multiple equilibria due to negotiation between the drug firm agents and the foreign sellers, the bias introduced leads to, g≠g^*and d≠d^*,the optimal sustainable development path is not Pareto efficient. The nature of the non optimality depends on the favour obtained by the abroad firms from the drug business staff expressed by s, such that:

If s=1, the tax rate is located at its highest level,  =(1+q) at the same time, the foreign firms which sells forgery drugs faces a high corrupt agent and try to obtain his favour for the firm to introduce low quality products in the market. The success of the negotiation depends on the value of the probability, if p is close to 1 then the optimal sustainable development path is reached because negotiations between some staffs and the non efficient firm fail, thus, g=g* and d=d* i.e sustainable path is reached. Otherwise, if p is close to 0, the optimal sustainable development path can’t be reached because negotiations between the staffs and the non efficient foreign firms achieved and then, both g and d converge respectively to 0 and to -∞.

If 0<s<1, then if s is high but q is not high enough, i.e 0<q<1 the whole forgery drugs are not introduced in the market, otherwise, if q=1, the most drugs received are forgery drugs, the development sustainable path can’t be reached because health alteration keeps increasing. The economy is unable to achieve his long-run optimal sustainable development path without health alteration. Indeed, there is almost no change of the drug business in regard to the tax rate set by the social planner mostly due to the fact that, incentives to improve the drugs quality are too low compare to the severity of control announced. Life expectancy duration is reduced.

If -1<s<0, this situation reveals a special economic policy deal and can be viewed in two different ways with respect to the impact on sustainable development, since we have, g≥g* and k≥k*, h<h* but d<d*. Two interpretations can be provided: First, the budget deserve to health care is higher than the average i.e k≥k* but not optimally used since, h<h* and d<d*. There is eviction on funds provided for health care improvement policy due to q=1. Second, the social planner discovered corruption activity and asks the drug seller firm to send back the forgery drugs ordered from abroad. Indeed, the surplus viewed is only the expectation for the drug seller to increase its activity’s quality beyond the threshold or the equilibrium. If the objectives to end corruption doesn’t achieves, the social planner must order the cease of their activities to punish corruption , if it is done, those firms may stop their activities because costs become higher than profit since high quality drugs deserve a higher investment. Therefore, drug quality control should deserve negotiations with high quality drug producers in cooperation with the government to ensure development sustainability occurrence since health state acts on the capacity to work, to earn money and to acquire human capital in the education sector, as well as to develop the country faster

Finally, multiple equilibria due to corruption come from poverty and the will to sell cheap products to poor consumers or to make them believe that they are doing something to take care of their health. Thus, poor countries should engage discussions on social security system existence or to choose some specific pharmaceutical companies to work with in order to reach the long run growth.

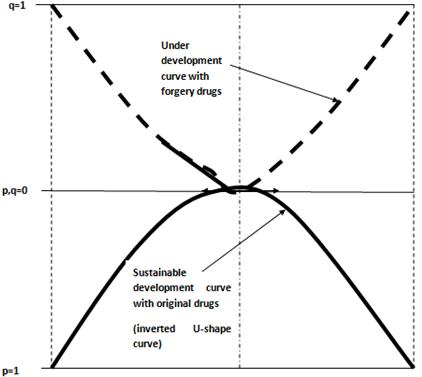
Figure 1. Summarizes the impact of drugs on development.

5. Conclusion

The article viewed the link between drugs and health and proved that forgery drugs lead to health alteration, thus retard development because it is a fundamental component of sustainability. The article dealt with corruption to highlight the fact that forgery drugs entry is caused by corrupted business which look for profit increase more that the agents’ security. Therefore, optimal program results led the social planner fix a tax on forgery drugs import to establish optimality and increase control on forgery products in the country. When all conditions are filled development emerge described by an inverted U-shape. (see figure 1 for summary of the analysis). Since health state depends on drugs quality, thus forgery drugs leads to health alteration, thus to under development. In contrast, original drugs, improve health, thus describes development like an inverted U-shape curve (Kuznets, 1955; Stockey, 1998; Smulders, 1995)

References

1. Aghion, P. and Howitt, P., 1992. A Model of Growth through Creative Destruction. Econometrica 60, 2, 323-351.
2. Kenneth, A. J., 1962. The Economic Implications of Learning by Doing, Review of Economic Studies 29, 155-173.
3. Azariadis, C. and Drazen, A., 1990, Threshold externalities in economic development, Quarterly Journal of Economics, 501-526.
4. Banuri, S. and Eckel, C., 2012, Experiments in Culture and Corruption, World Bank Policy Research
5. Bardhan, P., 1996, The Economics of Corruption in Less Developed Countries: A Review of Issues, Center for International and Development Economics Research, Working Paper No. C96-064, University of California, Berkeley: February.
6. Barro, R. J., 1996. Determinants of Economic Growth: A Cross-Country Empirical Study. National Bureau of Economic Research, working paper no.5698, August.
7. Barro, R. J. and Sala-i-Martin, X., 1995. Economic Growth. New York, McGraw Hill.
8. Barro, R. J. and Sala-i-Martin, X. 1991a, Convergence across States and Regions, Brookings Papers on Economic Activity, Economic Studies Program, The Brookings Institution, vol. 22(1), pages 107-182.
9. Caballe, J. and Santos, M., 1993, Journal of Political Economy, vol. 101, issue 6, pages 1042-67
10. Cass, D., 1965. Optimum Growth in an Aggregative Model of Capital Accumulation. Review of Economic Studies 32, 233-240.
11. Docquier, F., and De la Croix, D., 2012, Do Brain Drain and Poverty Result from Coordination Failure?, The Journal of Economic Growth, 17(1), 1-26.
12. Domar, E. D., 1946, Capital Expansion, Rate of Growth and Employment, Econometrica, 49, p.14-33.
13. Ehrlich, I. and Francis T. L., 1991. Intergenerational Trade, Longevity, and Economic Growth. Journal of Political Economy, 99 (5), 1029-1059.
14. Galor, O. and Weil, D.;2000, Population, Technology, and Growth: From Malthusian Stagnation to the Demographic Transition and Beyond." American Economic Review 90 (4): 806–28.
15. Grossman, G. M., and Helpman, E., 1991. Innovation and Growth in the Global Economy. Cambridge MA, MIT Press.
16. Harrod, R. F., 1939, An Essay in Dynamic Theory, Economic Journal, 49, p.14-33
17. Koopmans, T. C., 1965. On the Concept of Optimal Economic Growth, in The Econometric Approach to Development Planning, Amsterdam, North Holland.
18. Gideon Y., Rosin, O. and Tobol, Y., 2009, Junk-food, home cooking, physical activity and obesity: The effect of the fat tax and the thin subsidy. Journal of Public Economics, 93 (5), 823-830.
19. Lucas, R. E., Jr., 1988. On the Mechanics of Economic Development. Journal of Monetary Economics 22 (1), 3-42.
20. Meltzer, D., 1995, Mortality Decline, the Demographic Transition, and Economic Growth. Unpublished, University of Chicago.
21. Mulligan, C. B and Sala-i-Martin, X., 1993, Transitional Dynamics in Two-Sector Models of Endogenous Growth, The Quarterly Journal of Economics, MIT Press, 108(3), 739-73
22. Qian, R., 2012,Why do some countries default more often than others ? The Role of Institutions,Policy Research working paper; no. WPS 5993.
23. Ramsey, F., 1928. A Mathematical Theory of Saving. Economic Journal 38, 543-559.
24. Rebelo, S., 1991, Long-Run Policy Analysis and Long-Run Growth. Journal of Political Economy 99, 3, 500-521.
25. Romer, P. M., 1986. Increasing Returns and Long-Run Growth, Journal of Political Economy 94, 5, 1002-1037.
26. Romer, P. M., 1987. Growth Based on Increasing Returns Due to Specialization, American Economic Review 77, 2, 56-62.
27. Romer, P. M., 1990. Endogenous Technological Change, Journal of Political Economy 98, 5, S71-S102.
28. Rosen, S., 1988. The Value of Changes in Life Expectancy, Journal of Risk and Uncertainty 1, 3, 285-304.
29. Schumpeter, J. A., 1934. The Theory of Economic Development, Cambridge MA, Harvard University Press.
30. Sheshinski, E., 1967. Optimal Accumulation with Learning by Doing, in Karl Shell, ed., Essays on the Theory of Optimal Economic Growth. Cambridge MA, MIT Press, 31-52.
31. Stockey, N., 1998, Are There Limits to Growth?, International Economic Review, 39, 1-31.
32. Solow, R. M., 1956. A Contribution to the Theory of Economic Growth, Quarterly Journal of Economics 70 (1), 65-94.
33. Stokey, N., L., 1988, Learning by Doing and the Introduction of New Goods, Journal of Political Economy, 96 (4), 701-717.
34. Swan, T. W., 1965. Economic Growth and Capital Accumulation, Economic Record 32, 334-361.
35. Uzawa, H., 1965. Optimal Technical Change in an Aggregative Model of Economic Growth, International Economic Review 6, 18-31.